Internet Encyclopedia of Science
GEOMETRY
A  B  C  D  E  F  G  H  I  J  K  L  M  N  O  P  Q  R  S  T  U  V  W  X  Y  Z

                   • HOME
• ABOUT
• CATEGORIES
• USE OF TEXT AND IMAGES
• NEWSLETTER

  



unilluminable room problem


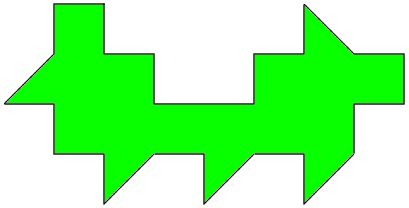
unilluminable room
Imagine an L-shaped room in which Amy is standing near one corner holding a match. If Bob stands round the corner, he can see light from the match because a light ray can bounce off the two opposite walls. This is true wherever Bob stand in the room: the whole room is illuminated by the one match. Would this be true for a room of any shape, or is there at least one room that is so complicated that there's somewhere inside it that light from the match never reaches?

This problem was first asked by Ernst Strauss in the 1950s. Nobody knew the answer until 1995, when George Tokarsky of the University of Alberta showed that the answer is "yes" – there is a room that is not completely illuminable.1 His published a floor plan of a room with 26 sides – the least-sided unilluminable room currently known. But a mystery remains. The room Tokarsky found contains one particular place where the match can be held which leaves part of the room dark. But if the match is moved slightly, the whole room is lit up again. Is there a room so fiendishly complicated that wherever the match is held there are some places that its light can never reach? For the moment we remain in the dark ...


References

  1. Tokarsky, George W. "Polygonal Rooms Not Illuminable from Every Point." American Mathematical Monthly, 102(10): 867-879, 1995.
  2. Stewart, Ian. "Unilluminable Rooms." Scientific American, 275(2), 100-103 (Aug. 1996).

Related category

   • GEOMETRY


Also on this site:

Encyclopedia of Alternative Energy & Sustainable Living
Encyclopedia of History




BACK TO TOP