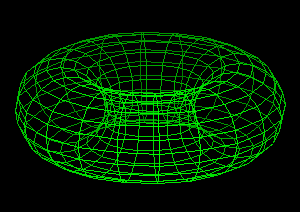
A torus is the two-dimensional surface of a doughnut or inner-tube shape; the word comes from the Latin for "bulge" and was first used to describe the molding around the base of a column. One way to think of a torus is as a surface of revolution obtained by rotating a circle around an axis that lies in the plane of the circle but doesn't intersect the circle.

 

Small circles can be drawn around the torus with radii equal to that of the generating circle, and they are called meridians. Circles of varying radii that go around the hole or center of the torus on parallel planes are called parallels. Both meridians and parallels on a torus are infinite in number.

 

The volume of a torus is π ²r ²d and its surface area is 4π ²rd, where r is the radius of the circle and d the distance of its center from the line.

 

In the general case, where the shape being so rotated is any closed plane curve, the resulting surface is called a toroid. Although, as said above, the usual torus in three-dimensional space is shaped like a doughnut, the concept of the torus is extremely useful in higher dimensional space as well.