Agnesi, Maria Gaetana (1718–1799)

Maria Agnesi.
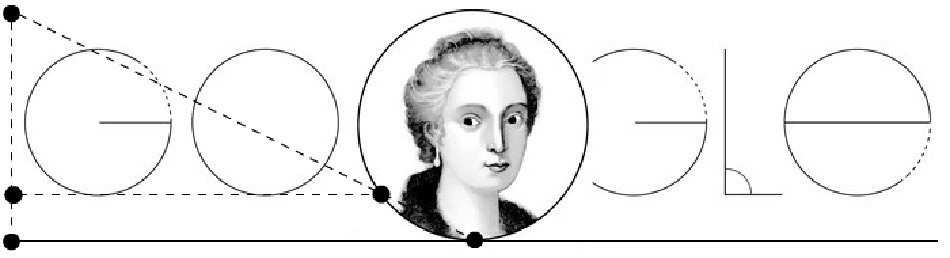
A Google 'doodle' on 16 May 2014 celebrated the 296th birthday of Marie Agnesi.
Maria Agnesi was an Italian mathematician and scholar whose name is associated with the curve known as the Witch of Agnesi. Born in Milan, Maria was one of 21 children of a three-times-married professor of mathematics at the University of Bologna. A child prodigy, she could speak seven languages, including Latin, Greek, and Hebrew by the age of 11, and was solving difficult problems in geometry and ballistics by her early teens. Her father encouraged her studies and her appearance at public debates. However, Maria developed a chronic illness, marked by convulsions and headaches, and, from the age of about 20, withdrew socially and devoted herself to mathematics. Her Instituzioni analitiche ad uso della gioventu italiana, published in 1748, became a standard teaching manual and, in 1850, she was appointed to the chair of mathematics and natural philosophy at Bologna. Yet she never fulfilled her early promise in terms of making new breakthroughs. After the death of her father in 1752, she moved into theology, and, after serving for some years as the directress of the Hospice Trivulzio for Blue Nuns at Milan, joined the sisterhood herself and ended her days in this austere order.
The famous curve that bears her name had been studied earlier, in 1703, by Pierre de Fermat and the Italian mathematician Guido Grandi (1671–1742). Maria wrote about it in her teaching manual and referred to it as the aversiera, which simply means to turn. But in translating this, the British mathematician John Colson (1680–1760), the fifth Lucasian Professor of Mathematics at Cambridge University, confused aversiera with avversiere which means witch, or wife of the devil. And so the name of the curve came down to us as the Witch of Agnesi. To draw it, start with a circle of diameter a, centered at the point (0, a/2) on the y-axis. Choose a point A on the line y = a and connect it to the origin with a line segment. Call the point where the segment crosses the circle B. Let P be the point where the vertical line through A crosses the horizontal line through B. The Witch is the curve traced by P as A moves along the line y = a. By a happy coincidence, it does a look a bit like a witch's hat! In Cartesian coordinates, its equation is:
y = a3/(x2 + a2)

