Stephen Hawking was an English theoretical astrophysicist and cosmologist famed almost equally for his work on black holes and the origin of the universe (propagated to a vast lay audience through his non-technical books, including A Brief History of Time and The Universe in a Nutshell) and the fact that he survived for five decades with motor neuron disease, an affliction with which he was diagnosed as a postgraduate at Oxford.

 

From 1980 to 2009 he held the Lucasian Chair of Mathematics at Cambridge University. He supplied a mathematical proof, along with Brandon Carter, W. Israel, and D. Robinson, of John Wheeler's "No-Hair Theorem" – namely, that any black hole is fully described by the three properties of mass, angular momentum, and electric charge. He also showed that black holes, especially tiny ones, can effectively evaporate as a result of the production of particle-antiparticle pairs in their vicinity, and, together with Ian Moss. has derived a "no-boundary" solution for the origin of space and time in the Big Bang.

 

Hawking radiation

 

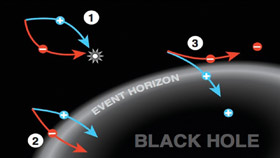In case (1), the particle-antiparticle pair annihilate before either component can fall into the black hole. In case (2), both components fall into the black hole, but in case (3) only one member of the pair is swallowed while the other escapes and gives rise to Hawking radiation.

 

Hawking radiation is a process, first theorized by Stephen Hawking, by which a black hole can apparently evaporate. When virtual particles are produced in the vicinity of a black hole, it is possible for one member of the matter-antimatter pair to be pulled into the black hole while the other escapes into space. The particle that falls into the black hole negates some of its mass and so the black hole shrinks a little. This makes it look as if the particle that escaped into space has come from the black hole. Hawking radiation would be particularly important in the case of miniature black holes, which might explode in this way.