gravitational waves
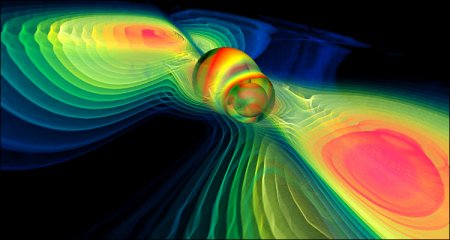
Figure 1. Computer model of the gravitational waves coming from the collision of two black holes. Image: MPI for Gravitational Physics/W.Benger-ZIB.

Figure 2. The LIGO facility at Livinston, Louisiana.

Figure 3. Artist's impression of how the white dwarf pair in J0651+2844 would radiate spirals of gravitational waves.
Gravitational waves are vibrations in the fabric of spacetime which travel at the speed of light and are predicted by Einstein's general theory of relativity. The most intense gravitational waves, resulting from large masses undergoing high accelerations, are expected to be produced when black holes or neutron stars collide, by supernovae, or by events at the formation of the universe itself (Figure 1).
The first detection of gravitational waves was made on 14 September 2015 by LIGO (Laser Interferometer Gravitational-Wave Observatory), which is operated jointly by CalTech and MIT. The source of these waves turned out to be two black holes, with masses of 29 and 36 times that of the Sun, that had been orbiting around each other and then collided. In the final fraction of a second before they merged these black holes released more than 50 times the power of all the other stars in the observable universe combined.
Prior to this, indirect evidence for their existence aome from radio-wave observations of the only known binary star in which both components are neutron stars, and also from visible-light observations of a pair of white dwarfs. A gradual, minuscule increase in the orbital period of these systems can be accounted for exactly if energy is being lost by gravitational waves at the rate predicted by general relativity.
Physics of gravitational waves
In general relativity, massive objects affect the way that spacetime curves. Picture spacetime to be like the elastic skin of a trampoline. A person standing on the trampoline creates a hollow in the rubber sheet, just as a mass in spacetime causes a dip to form in the spacetime around it. If the person bounces up and down on the trampoline, she causes vibrations to travel out across the sheet. In the same way, says general relativity, a massive object that suddenly shifts will create ripples in the fabric of spacetime – ripples known as gravitational waves.
The existence of gravitational waves was first talked about by physicists, such as the Englishman Oliver Heaviside and the Frenchman Henri Poincaré. In a sense, the idea of gravitational waves was implicit in the 1905 special theory of relativity, with its finite limiting speed for the transfer of mass, energy, or information. No effects, including those of gravity, it insisted, can get from one place to another faster than the speed of light. But the exact equations describing gravitational waves weren't revealed until general relativity came along. In 1916, and in more detail in a 1918 paper, Einstein showed that when a mass accelerates – in other words, changes its state of motion – it can't help but give rise to time-varying gravitational fields that travel away from the source at light-speed as undulations in the surface of spacetime.
Anything with mass, or any collection of things with mass, spawns gravitational waves when it changes shape, or spatial arrangement, or velocity. Think of these waves coming about in the same way that electromagnetic waves do. An electrically-charged moving object gives off electromagnetic waves in proportion to its charge and acceleration. Likewise, a moving mass generates gravitational waves in proportion to its mass and acceleration. There's just one important difference. Newton's third law of motion says that the acceleration of a mass in one direction must be accompanied by an acceleration of another mass in another direction, with momentum (mass times velocity) in both directions being equal. This means that the other mass generates gravitational waves as well, so that the waves of the two masses tend to cancel out. Because the masses aren't in the same place, however, the cancellation is never perfect. The amount of gravitational radiation that gets away depends on the arrangement of the masses, measured by what's called the quadrupole moment. A totally symmetrical object, like a soccer-ball shape, has zero quadrupole moment, whereas something very unsymmetrical, like an American football-shape, has a large quadrupole moment, at least for rotation around its short axis.
If a mass undergoes some sudden change, the resulting gravitational waves will take the form of a short pulse, much like the first big ripple produced after dropping a rock into a still pond. In the case of a periodic change, the wave will be sustained, like the carrier wave for a broadcast radio signal. In either case, the amplitude, or height, of the wave will steadily fall as the distance from the source increases.
Gravitational waves are transverse, as are light and water waves, which means they vibrate at right angles to the direction in which they're traveling. To appreciate how this affects their interaction with matter, suppose four masses are arranged at the four points of a compass in the horizontal plane, and a gravitational wave passes through the plane from above. At one point, the distance between the north-south masses is decreased, and the distance between the east-west masses is increased. A half wavelength later, the reverse is true. If the gravitational wave passes through the masses along the direction of the plane, say in the east-west direction, it has no effect on the masses in the direction of its motion. The east-west masses remain fixed in position, while the distance between the north-south masses increases and decreases as the wave passes through the plane.
If general relativity is to be believed, the universe should be awash with gravitational waves. Yet Einstein never took them seriously. He tended to think of them as mere mathematical artifacts – things that were possible in theory but that, in reality, would never be strong enough to have measurable effects. It was a view shared by most of his colleagues, among them Arthur Eddington, whose eclipse measurements gave general relativity such a powerful boost. Derisively, Eddington commented, "Gravitational waves propagate at the speed of thought."
But what if they existed and could be picked up and recorded? Then they might open up a whole new window on the cosmos. In the 1950s, the first giant radio telescopes began giving astronomers an astonishing view of the universe beyond that available in ordinary light. Around the same time, theoreticians managed to show that gravitational waves are, at least in principle, detectable.
In principle. It's important to get the scale of the observational problem in perspective. Imagine that several thousand light-years away some stellar cataclysm occurs that generates the gravitational equivalent of a tsunami. By the time that once-mighty wave has reached our stretch of the galactic shore its influence on spacetime, as it passes by, will be to cause a distance as large as that between the Earth and the nearest star (after the sun) – about 40 trillion miles – to alter temporarily by about the width of a human hair. An instrument sensitive enough to detect that would be able to sense a change in the Earth-sun distance of about the width of an atom: roughly a millionth the diameter of a proton per meter. Any way you slice it, it's asking a lot of our engineering ingenuity.
The detection problem goes back to the feebleness of gravity. Even when large, sudden movements of huge amounts of matter are involved, the waves produced are bound to be fantastically weak by the time they've made their way to us across many light-years of space. A gravitational wave arriving on Earth will alternately stretch and shrink distances, but on a mind-numbingly small scale.
Yet, the promise of what gravitational wave astronomy might tell us about the universe is too great to allow technical difficulties to stand in the way if we can help it. The most powerful gravitational waves will produced by the most violent events. Many of these are expected to involve matter in its most condensed state, in the form of black holes and neutron stars – bizarre denizens of the cosmos unknown in Einstein's day. Great surges of gravitational radiation are thought likely to come from the implosion of a stellar core at the heart of a supernova to form a black hole or a neutron star, by the swallowing of a neutron star by a black hole, or by the collision and merging of two black holes. Observing gravitational waves could give us an independent means of estimating cosmological distances and of peering back to the earliest moments when space and time came into being. They might also reveal phenomena of which we previously had no inkling.
Almost all our information about the cosmos at present comes in the guise of electromagnetic waves – visible, radio, infrared, ultraviolet, X-ray and gamma-ray. A smattering arrives by way of cosmic rays and neutrinos. But that's about it. Gravitational waves offer an entirely new vista on what's out there, especially at the high-energy, fast-changing end of the phenomenological scale. And, although their detection poses enormous challenges, they do have at least one advantage over their electromagnetic counterparts. Whereas light, radio waves and so forth, are affected by intervening matter (light is strongly absorbed by interstellar dust, for example), gravitational waves are expected to pass unchanged through any material in their path and so be able to carry signals with absolute clarity across the vast reaches of space.
Gravitational wave detectors
The late 1960s saw the first gravitational wave detector take shape at the University of Maryland at College Park. Its creator, Joseph Weber, then caused a minor sensation by claiming a couple of years later that his instrument had come up with the goods.
Weber's detector used two huge (1.5-ton) solid aluminum cylinders to which piezoelectric crystals were wired. These two bars were placed at separate locations in order to sift out random noise and other false readings, the idea being that only signals that affected both bars would be recorded. If a suitable gravitational wave came along it would trigger the so-called fundamental longitudinal mode of the cylinder, whose natural frequency was around 1,600 Hz (cycles per second), causing the cylinder to deform like a rubber bung that's alternately stretched and squeezed along its lengthwise axis. This frequency was chosen because it's in the range that astrophysicists had predicted for the short pulses of gravitational waves expected from supernovae. In Weber's setup, the piezoelectric crystals bonded to the surface of the cylinder were intended to respond to any deformations by producing electrical voltages that could be read out.
Not long after firing up his rig, Weber saw what he took to be strong evidence for gravitational waves; the number of periodic signals detected, he reported, far outweighed the amount of noise, and, when the detector was pointed for optimum reception of waves from the center of the galaxy, the recorded observations peaked.
Yet Weber's claims ran into a wall of skepticism. For one thing, it seemed that his equipment was nowhere near sensitive enough to be picking up the number of hits he was talking about. To give some examples, the strongest gravitational waves coming from a binary system in which there are two collapsed objects, such as neutron stars or black holes, orbiting close to each other, would distort a gravitational wave detector by about one part in 1020 (a hundred million trillion). The supernova that occurred in the Large Magellanic Cloud (a satellite galaxy of our own) in 1987 (see supernova 1987A) – the nearest, brightest such event in more than three centuries – might have managed a distortion of one part in 1019. And an even closer supernova in the disk of the Milky Way would push that distortion up by another order of magnitude to about one part in 1018. Yet one part in 1018 was the supposed limit of sensitivity of Weber's apparatus. His positive readings couldn't possibly have been due to nearby supernovae because none had been confirmed by other means; in fact, the last recorded supernova in the Milky Way was the one seen by Kepler in 1604. Either gravitational waves in general were at least a thousand times more powerful than anyone had predicted, enabling Weber to detect them coming from sources less violent than supernovae. Or, something was wrong with his experiment.
In the end, the latter turned out to be the case. Weber's machinery was riddled with so much noise that any genuine gravitational wave signal would have been hopelessly masked. The biggest source of this background chatter was the incessant thermal vibrations of the atoms in the metal cylinders. These alone were enough to cause a longitudinal oscillation a hundred times stronger than any that might stem from a gravitational wave.
More experiments followed around the world using the so-called resonant antenna method that Weber had pioneered. All these strived to improve sensitivity and cut noise in various ways, most importantly by cooling the test masses to temperatures close to absolute zero so that thermal motions were kept to a minimum. Another problem was the noise of the electrical transducers and amplifiers used to process the gravitational wave signal. Modern detectors have done away with piezo crystals in favor of cavity resonators or coils whose electric properties are changed by the deformation of the test mass. To boost the signal noiselessly, they use SQUIDs (superconducting quantum interference devices) in which quantum effects do the job of amplification. And, in the latest bar detectors, the whole test mass is suspended as a pendulum to isolate it as much as possible from seismic and other external disturbances. Aided by all these tricks, today's resonant detectors can sense deformations of 10–19 meter, comfortably giving them the sensitivity needed to detect the strongest of gravitational waves. So far they've heard nothing. But if there's another supernova in our galactic neighborhood, like the one in 1987, or better still in the Milky Way itself, that might change. Efforts, too, are continuing to improve the sensitivity of resonance antenna.
At the same time, scientists have realized there's another way to bring gravitational waves into view. Known as laser interferometry, it exploits an arrangement similar to that of the famous Michelson-Morley experiment. A laser interferometer gravitational wave detector (LGD) doesn't measure the distortion of masses as such. Instead, it looks for a change in length of a pair of long pathways at a right angles to each other using a laser beam.
The idea of applying laser interferometry to the problem of detecting gravitational waves was dreamt up in the late 1960s by Ranier Weiss of the Massachusetts Institute of Technology. A decade later, encouraged by the physicist Kip Thorne (who, as a student at Princeton, had been a fan of Weber's work), a well-funded research effort on LGDs sprang up at Caltech. Then, in 1983, the Caltech and MIT groups got together and began what evolved into one the biggest efforts to date to detect gravitational waves. Called LIGO (the Laser Interferometer Gravitational-Wave Observatory) it's been built at a cost of $365 million and has recently kicked off its scientific work in earnest (Figure 2).
LIGO consists of two identical facilities: one at Hanford, on the plains of south-central Washington state, the other at Livinston, Louisiana, in a forest near Baton Rouge. At each location is a strange L-shaped building with two skinny arms two and half miles long. At the vertex of the L is the nerve center of the operation, where a flashlight-sized, ultrastable laser beam is split and sent down each arm inside a four-foot-diameter, stainless steel vacuum tube. At the far end of the arm a delicately suspended mirror reflects back the laser light, and the two returning beams are recombined and fed to a photodetector. A passing gravitational wave will slightly compress one tube while simultaneously stretching the other, causing one mirror to move slightly forward, and the other slightly back. The effect of that tiny movement is to cause a slight phase shift in the laser light traveling along the two arms; it's this phase shift that the detectors are designed to measure. Having two installations in different parts of the country helps scientists see past the extraneous noise in the system. Events detected by LIGO are only be considered potentially valid if they happen within 10 milliseconds of each other – the maximum time taken by a gravitational wave to travel the 1,890 miles between Hanford and Livingston.
LIGO can search for gravitational waves with widely differing frequencies. A bar detector has to be specially constructed, much like a tuning fork, for each frequency. Ultimately, though, it's hoped to link all the world's major gravitational wave observatories, of whatever type, into a global network, so that as much information as possible can be extracted from any signals received. Comparing the times when a wave passes different detectors, for example, will give information about the direction of the source in the sky. Accurate timing, strength and frequency data will also help shed light on the physical processes that created the waves.
Ground-based gravitational wave detectors are expected to make huge strides over the coming decade or so. Yet these facilities are inevitably subject to vibrations of Earth itself, rendering them useless for picking up low-frequency waves. This type of gravitational wave is thought to be created when a supermassive black hole at the heart of a galaxy swallows a star. So, if we hope to be able to "see" the spacetime ripples from such an event we to need put our gear where no planetary rumblings can effect it – in space. Enter LISA (the Laser Interferometer Space Antenna). This project, being developed jointly by NASA and the European Space Agency, will involve placing three identical satellites in orbit in the configuration of a triangle with sides three million miles long. Each LISA satellite will look like a ring with a Y-shaped core, containing a laser and telescope assembly to allow it to link up with its two partners. The test masses will be cubes one and a half inches on a side, floating freely inside the spacecraft. A prototype system is expected to be launched first, followed by the full LISA array in about 2018.
Hulse-Taylor pulsar
Even more gravitational waves were detected directly, scientists no longer seriously doubted they exist. Much of that optimism stemmed from a discovery made in 1974 by two radio astronomers at the University of Massachusetts at Amherst, Joseph Taylor and his graduate student Russell Hulse (both now at Princeton). Taylor and Hulse had been using the giant Arecibo radio telescope, in Puerto Rico, to search for new cosmic radio sources when they picked up a highly regular pulsed signal coming from a point in the sky just a few degrees away from the bright star Altair. Seventeen times a second the little radio beacon pulsed, as regular as clockwork. Taylor and Hulse knew right away that they'd found a new pulsar (a fast-spinning, highly-magnetized neutron star) – an object that would be catalogued as PSR 1913+16.
Another pulsar added to the inventory isn't in itself such big news: over 700 have been identified since the first came to light in 1967. But as the two astronomers studied their new discovery more closely, they uncovered something very unusual indeed: there was a systematic variation in the arrival time of the pulses. Sometimes, the pulses came a little sooner than expected – up to three seconds sooner. Sometimes they came a little later, by up to the same amount. These variations happened in a smooth and repetitive manner, with a period of 7.75 hours. Taylor and Hulse now knew they'd captured a much rarer animal. This was no pulsar wandering through the galaxy alone. It had a companion, a stellar partner. And these two stars were engaged a rapid pas de deux.
Seen in the context of a binary system, the changing arrival times of the pulses make sense. When the pulsar is on the side of its orbit closest to the Earth, the pulses arrive earlier that they do when the pulsar is on the side furthest away from us. The difference of three seconds corresponds to the time taken for radio waves to travel the one-and-a-half-million-miles from one side of the orbit to the other. Further observations showed the orbits of the two stars around their common center of gravity to be quite eccentric, or stretched out. At minimum separation, known as periastron, the pulsar and its companion are only about one solar radius apart; when furthest apart, at apastron, slightly more than two suns could be fitted in the gap between them.
The beauty of a binary system is that the mutual motions of the two components reveal the secret of their masses. Very quickly Taylor and Hulse were able to establish that the pulsar and its dance partner have an almost identical mass: about 1.4 times that of the sun. Since neither star showed up on optical pictures, the conclusion was inevitable and extraordinary. The pulsar's companion was also a collapsed object – another neutron star. Taylor and Hulse had discovered the first binary neutron star system.
Astronomers had long speculated that such systems must exist. And they also knew, that if two dense, heavyweight objects were found, whipping around each other in a tight, fast orbit, they'd serve as an ideal natural laboratory for testing various predictions of general relativity. The existence of the pulsar was a godsend because the pulsing of its radio emission were like the ticks of an accurate clock. The discoverers of PSR 1913+16 had uncovered a gravitational gold mine.
According to Einstein's theory, the stronger a gravitational field is, the slower time will run in it. Since the orbit of PSR 1913+16 around its companion is elliptical, the two stars are closer together at some times than at others, so the gravitational field alternately strengthens at periastron and weakens at apastron. When the stars are closer together, near periastron, the gravitational field is stronger, so, according to general relativity, the passage of time should slow and therefore the interval between pulses should lengthen. Likewise, the pulsar clock ought to regain time when it's traveling in the weakest part of the field around apastron. Taylor and Hulse's measurements completely vindicated Einstein's predictions on this score.
They also explained an effect that would have had Newton completely baffled. Recall the anomalous movement, or advance, of Mercury's perihelion – the extra 43 arc-seconds per century by which it drifts around the Sun above and beyond what Newtonian theory expects (see advance of perihelion). In the strange realm of the Hulse-Taylor pulsar, spacetime is vastly more warped, with the result that the periastron advance of the component stars makes Mercury's aberrant motion look like a trivial accounting error. PSR 1913+16's point of closest approach to its partner shifts by an astounding 4.2 degrees per year; in other words, its periastron advances in a single day by the same amount as Mercury's perihelion advances in a century. Remarkable though this figure seems, it's exactly in line with the bookkeeping of general relativity.
These data from the Hulse-Taylor system, showing the slowing of time and the periastron drift, merely confirmed other observations made previously by astronomers and physicists that supported Einstein's view on gravity. But another effect was seen for the first time thanks to careful studies of the pulsar's metronomic ticking. In 1983, Taylor and his colleagues reported that there'd been a systematic shift in the observed time of periastron relative to that expected if the average orbital separation between the two stars had remained constant. Data taken in the first decade after the discovery showed a decrease in the orbital period of about 76 millionths of a sec per year. By 1982, the pulsar was arriving at its periastron more than a second earlier than would have been expected if the orbit had remained constant since 1974. Put simply: its orbit is decaying. For every circuit that PSR 1913+16 completes around its companion, its orbit shrinks by just over a tenth of an inch. And Taylor and Hulse knew exactly why.
Take two big masses that are close together, whirl them around their common center of gravity at high speed, and general relativity is clear about what will happen. The orbital energy of the masses will gradually radiate away. They'll give off gravitational waves and, as they do so, their orbits will get smaller and smaller. Ten years – and now ten more years – of watching PSR 1913+16 have shown its orbital period to be dropping at just the rate expected due to loss of orbital energy by gravitational radiation. And while that isn't proof of the existence of gravitational waves, it's a very persuasive sign. It was evidence enough, at any rate, to persuade the Nobel committee that Taylor and Hulse should receive the 1993 Nobel Prize in Physics.
In time, the pulsar and its mate will come so close together that they may
tear each other apart in their ferocious gravitational embrace. Barring
that, a collision is inevitable, some 240 million years from now. A final,
powerful burst of gravitational waves will mark the event and, if our descendants
are still around and listening, be recorded as a sharp spike in detectors
here on Earth.
J0651+2844
In Aug 2012, astronomers announced that they had found evidence for gravitational waves in the visible light coming from a pair of white dwarfs orbiting each at a very close distance (Figure 3). In this system, known as J0651+2844, which lies 3,000 light-years from Earth, the white dwarfs are just one-third the distance from the Earth to the Moon apart, and complete an orbit around each other every 13 minutes. Since their orbital period was first measured in 2011, the same team has been closely monitoring it for any slight changes – a feat that is possible because the stars pass in front of each other as seen from our vantage point so that the total light from the system varies every 6 min or so. In 13 months of observation, the eclipse time shifted back by 6 seconds – an amount explainable because of a drain in the system's orbital energy in the form of gravitational waves.{1}
Future of gravitational wave astronomy
Powerful gravitational waves are given off by binaries in which a black hole orbits a neutron star, or two black holes circle around each other. Observing them may confirm a curious fact that's emerged from computer simulations. These simulations suggest that no matter how a black hole forms or is disturbed, by infalling material, collision with another object, or orbital interactions with a companion, it will "ring" with a unique frequency – its natural mode of vibration. Detecting this unique signature in the form of gravitational waves would not only tell scientists that their theories were on the right track but also supply valuable information about the black hole's size and how fast it's spinning.
Black holes of the supermassive variety, thought to exist at the heart of many galaxies, are expected to leave another characteristic fingerprint of gravitational waves as they dine on the occasional star that comes too close to their lair. Finding such waves would supply direct proof that these dark dwellers of the galactic deeps are real. For physicists, all such studies of gravitation in highly relativistic settings are important because they afford a way to test general relativity in extreme situations (the so-called strong-field limit), where Einstein's theory isn't merely a small correction to Newtonian gravity. Watching for gravitational waves from regions where the curvature of spacetime is pronounced opens up the possibility of fundamentally new science.
Many researchers relish the prospect of what gravitational wave astronomy may do for our understanding of supernovae. A massive star faces a violent death when it runs out of nuclear fuel. The center of the star can then no longer support itself against gravitation and collapses under its own weight. At the same time, large parts of the star explode into space. After the explosion, a neutron star, or in the case of a very massive star, a black hole, remains. A fine scientific reward will follow the detection of gravitational waves from supernovae. Then we'll be able to understand the final stages of a star's evolution, and maybe actually watch neutron stars and black holes being born.
One supernovae or so per century is a typical galaxy's standard allotment. Since we're likely to have a long wait between these stellar eruptions in our own galaxy, we'll need to build bigger and more sensitive detectors that will let us see further out into the universe. There's even the possibility that, with future technology, we'll be able to pick up the faint gravitational waves left over from the Big Bang. That might also tell us something about the future of the universe. In contrast to electromagnetic radiation, it isn't fully understood what difference the presence of gravitational radiation has on a cosmological scale. It may be that a strong enough sea of primordial gravitational radiation, with an energy density exceeding that of the Big Bang electromagnetic radiation by a few orders of magnitude, would have an effect on the rate at which the universe is expanding.
Reference
1. Hermes, J. J., et al. "Rapid orbital decay in the 12.75-minute WD+WD binary J0651+2844". For publication in ApJ Letters. http://arxiv.org/abs/1208.5051.

